Welcome to Math 101, where great mathematicians are born! I will post intriguing activities, course announcements, and other exciting information here throughout the semester. Check back frequently. Below are links to some resources we may find useful in the course.
Math 101 – Spring 2019: Training Workouts
Below are all of the Training Workouts for Math 101 – Spring 2019 to date. For the most recent Training Workout and additional information see the Math 101 Home Page.
Welcome!
Training Workout #1 – due Tuesday, Feb 5, 2019
- Read the Course Syllabus linked to below. Let me know if you have any questions.
- Obtain and organize the following required items for the course. Bring the first two to class with you from now on.
- Problem Training Notebook – Record all of your work for problems that require it in this notebook.
- Must-Know Notebook – This can be a separate notebook or a section in the Problem Training Notebook.
- Training Log I will send you a link by email to share a spreadsheet with me for your Training Log. It is due once per week on Tuesday before 6:00pm, and it should cover everything you did for the problem solving course from Tuesday thru Monday inclusive of the previous week. See the sample spreadsheet for examples.
- Work on the problems from the ten problem set you were given in class. For each question do the following.
- Read the problem carefully and see if you can figure out what it is asking.
- If there is a term or word or math concept in the question that you don’t know or understand, mark it down to ask me in class if you can’t look it up and figure out what it says yourself.
- Try to answer as many of the questions as possible. However, your goal should not be to get the correct answer, but rather to be able to explain why your answer is correct. You may be asked to explain your solution at the start of class.
- Calculators are allowed on these problems.
Hand in just the answers you obtained on this set of problems on the official Assignment Answer Form from the link below. Label the form “Training Workout #1” and put your name on it. You can keep the questions. Show your work in your Problem Training Notebook.
Training Workout #2 – due Thursday, Feb 7, 2019
- Work on the problems from the team round problems you worked in class that you didn’t figure out. Try to answer as many of the questions as possible. However, your goal should not be to get the correct answer, but rather to be able to explain why your answer is correct. You may be asked to explain your solution at the start of class. Write any answers you get on the official answer sheet below.
- Save the other ten problem set (Warm Up 1) for another time.
Training Workout #3 – due Tuesday, Feb 12, 2019
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
- Work on the problems from the team round problems and Warm Up 1 that I handed out in class. Try to answer as many of the questions as possible. However, your goal should not be to get the correct answer, but rather to be able to explain why your answer is correct. You may be asked to explain your solution at the start of class. Write any answers you get on the official answer sheet below.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
Training Workout #4 – due Tuesday, Feb 19, 2019
- Write up your explanation for your solution to Part (a) of the Prove it! Admissions Test problem handout I gave you in class. You don’t have to solve Part (b) which is much, much, harder but I left it there as a Bonus problem in case you want to give it a try. Write up your solution and explanation in essay/paragraph form and hand it in on paper in class. You can type it or handwrite it. You can use blank paper or notebook paper, it should not be the official answer form below.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
Training Workout #5 – due Thursday, Feb 21, 2019
- Try to solve as many of the ten questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
Training Workout #6 – due Tuesday, Feb 26, 2019
- Read the instructions for Stars! by clicking here. Then play Stars! and try to beat all of the levels of the game, not by luck, but by figuring out how to win in every case (or prove that you can’t). Note that pressing “New Game” gives you a new game with the same moves available, but changing the difficulty changes what moves are available.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
Training Workout #7 – due Thursday, Feb 28, 2019
- Color the three HeeBGB’s whose generators are shown below. You should use a highlighter marker like we used in class and draw the fifth iteration. You can print the graph paper you need by clicking here. For part (c) choose your own generator as long as it is different from the ones in parts (a) and (b) and those we did in class. Hand in your colored results in class. Put your name at the top of the page. For part (c) draw your generator at the top of the page so I know what one you are trying to draw.
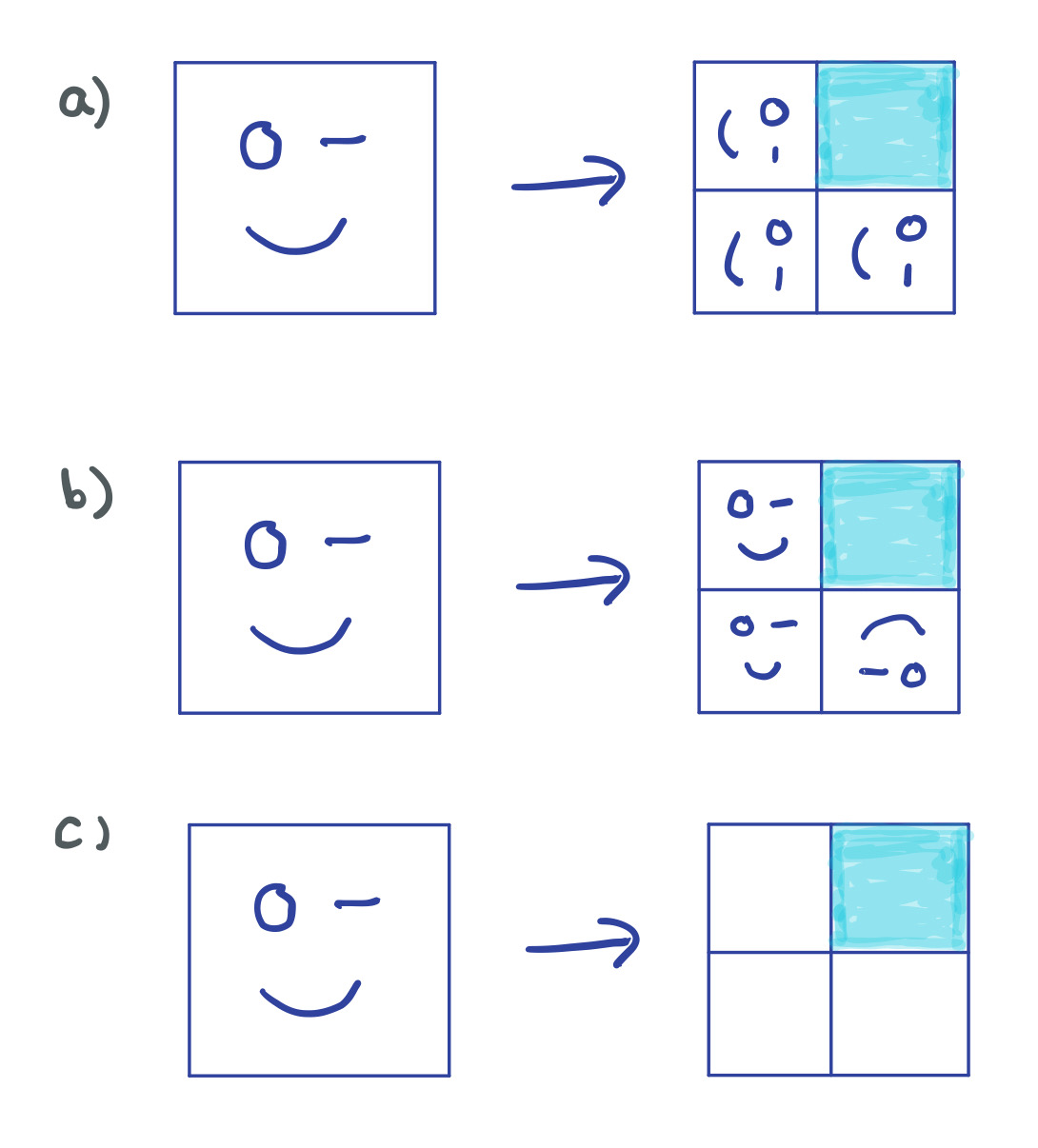
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
Training Workout #8 – due Tuesday, March 5, 2019
- Solve the mapping puzzle handout I gave you in class. You can print a copy for your scratch work, and hand in just your final answer on the original handout with your name on top.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
Training Workout #9 – due Thursday, Mar 7, 2019
- Try to solve as many of the ten questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spent in class since the start of the term if you are filling out the sheet for the first time.
Training Workout #10 – due Tuesday, March 19, 2019
- Solve the mapping puzzle handout I gave you in class. You can use one copy for your scratch work, and hand in just your final answer on the original handout with your name on top.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester.
Training Workout #11 – due Thursday, Mar 21, 2019
- Try to solve as many of the ten questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #12 – due Thursday, Mar 28, 2019
- Try to solve as many of the ten questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Bring your Rubiks Cube to class – we are going to start learning how to solve it.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #13 – due Tuesday, April 2, 2019
- Try to answer the questions on the handout I gave you in class. Don’t just hand in your answers, but rather write out an explanation of how you came up with them and hand that in. The hint I gave you on the handout is very helpful!
- Practice putting just to bottom four edge cubies in the correct location on your Rubik’s Cube – You will need to master that step first before you can advance to the next step.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #14 – due Thursday, April 4, 2019
- Try to solve as many of the ten questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Bring your Rubiks Cube to class – we are going to learn the next step of how to solve it.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #15 – due Tuesday, April 9, 2019
- Try to answer the question on the handout I gave you in class. Don’t just hand in your answer, but rather write out an explanation of how you came up with it and hand that in. The hint I gave you on the handout is very helpful!
- Practice putting the bottom eight edge cubies in the correct location on your Rubik’s Cube – You will need to master those two steps first before you can advance to the next step.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #16 – due Thursday, April 10, 2019
- Try to solve as many of the ten questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Bring your Rubiks Cube to class – we are going to learn the next step of how to solve it.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #17 – due Tuesday, April 16, 2019
- Review the Attendance Policy in the Syllabus – in particular keep in mind that after two missed classes, missing a class severely impacts your course grade. Most of what we do is in class, so attendance is a major component of your grade.
- Try to answer the ten questions on the handout I gave you in class. Hand in just your answers on the standard answer sheet below.
- Practice putting the twelve edge cubies in the correct location on your Rubik’s Cube – You will need to master that step before you can advance to the next step.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #18 – due Tuesday, April 23, 2019
- Try to answer the question on the handout I gave you in class. Don’t just hand in your answer, but rather write out an explanation of how you came up with it and hand that in.
- Practice putting the twelve edge cubies in the correct location on your Rubik’s Cube – You will need to master those two steps first before you can advance to the next step.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #19 – due Thursday, April 26, 2019
- Try to solve as many of the questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Bring your Rubiks Cube to class – we are going to learn the next step of how to solve it.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #20 – due Tuesday, April 30, 2019
- Try to figure out a winning strategy for TriX Game. You can play it online at my website by clicking here. Don’t just beat it at the easy levels. Instead figure out a method to beat any level no matter what number your goal is. You may be tested in class to see if your method works.
- Practice putting the twelve edge cubies in the correct location and orienting them correctly on your Rubik’s Cube – You will need to master those steps first before you can advance to the next step.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #21 – due Thursday, May 2, 2019
- Try to solve as many of the questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Bring your Rubiks Cube to class – we are going to learn the next step of how to solve it.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #22 – due Tuesday, May 7, 2019
- Try to solve the Enemies handout problem I gave you in class. Write up your explanations and answers on separate paper. Use complete sentences to explain your reasoning. Give examples where necessary.
- Bring the answers to the last assignment with you. We may go over some of those questions at the beginning of class.
- Practice solving your Rubik’s Cube – Ask me about the first point where you are stuck so we can get you unstuck.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Training Workout #23 – due Thursday, May 9, 2019
- Try to solve as many of the questions as you can on the handout I gave you in class. Hand in just your answers on the official answer form below.
- Bring your Rubiks Cube to class – we are going to learn the next step of how to solve it.
- Be sure to know all of the Must-Know facts. We may have a surprise quiz at any time at the start of class.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class.
Final Exam – Thursday, May 16, 2019 – 5:30-7:30PM
- Study all of your Must-Know Facts – we will have a longer-than-usual Must-Know quiz of problems using those facts.
- Bring your Must-Know and Problem Solving Notebooks – they will be graded and given back to you.
- Bring your Training Log up to date – log any hours you put in this semester. You can record hours we spend in class. It will be graded as part of your final grade.